Illustrative Mathematics
Box of Clay
What happens to a volume when you scale the dimensions of a rectangular prism? For this problem, a box of clay is increased in each dimension, with the intent to see if learners can generalize the result. The addition of physical models...
Mathematics Assessment Project
Fruit Boxes
Perfect for visual and hands-on learners, an engaging lesson prompts pupils to consider the different-sized boxes they can create from a piece of cardboard. They develop a model to determine the size of the box with the greatest volume.
Curated OER
Surface Area and Volume
Accompanying the lesson at www.shodor.org, this worksheet present learners with a series of questions about the volume and surface area of a rectangular prism and a triangular prism. Students predict how these values would change if the...
Curated OER
Similarity of Length, Area, and Volume
In this similarity worksheet, students determine the length, area and volume of similar figures. This three-page worksheet contains explanations, examples, and 20 problems. Answers are provided on page three.
Curated OER
Geometry Practice: Volume
In this volume worksheet, students find the volume of 6 solids. Students find the volume of rectangular and triangular prisms.
Curated OER
Lesson One: Perimeter, Area, Volume
Students explore perimeter, area and volume. Using geoboards, toothpicks, and marshmallows, students create specific shapes. They are directed to use formulas to find the volume, area, and perimeter of the created shapes. In groups,...
Curated OER
Area, Surface, and Volume
Ninth graders demonstrate application of area, surface, and volume. They apply formulas to a model of the classroom so they can determine the volume and surface area of the classroom.
Curated OER
Finding Volume
In this volume worksheet, students are given the equations for finding the volume of a cube and the volume of a prism. They solve two multi-part problems finding the volumes of different solids.
Curated OER
Perimeter, Area, Volume, and Surface Area
For this geometry activity students solve problems involving perimeter, area, volume, and surface area for various geometric shapes. The four page activity contains twenty-five problems. Answers key is provided.
Curated OER
Problem Solving - Use a Formula 21.7
In this formula activity, students complete a graphic organizer, answering the questions relating to: understand, plan, solve, look back. Students solve 2 problems. Houghton Mifflin test is referenced.
Curated OER
Twenty Volume Of Solids Problems
In this volume worksheet, students solve twenty volume of various solids problems. The solids examined include: cylinders, cubes, rectangular prism, including how the volumes of these figures change with increased in length. The...
Mathematics Assessment Project
Estimating Volume: The Money Munchers
Don't stuff money under your mattress. To find out why learners first complete a task determining how $24,000 in cash would affect the height of a mattress and whether this same amount would fit into a suitcase of given dimensions. They...
Concord Consortium
Swimming Pool II
Combine geometry and algebra concepts to solve a modeling problem. Young scholars consider the effect surface area has on volume. They write a cubic function to model the possible volume given a specific surface area and then determine...
Illustrative Mathematics
Christo’s Building
Hook your charges on how to solve a real-world art problem with mathematics by showing works of Christo. You can find eye-catching images on the Christo and Jeanne Claude webpage. Here, math learners help Jean Claude and Christo prepare...
Curated OER
Surface Area of Prisms
Students compute surface area and volume of prisms. In this surface area lesson, students find the surface area and volume of rectangular and triangular prisms. Independently, students use their computers to determine the volume,...
Curated OER
Prisms and Pyramids Homework
In this surface area and volume of prisms instructional activity, students identify the requested measurement of each of 10 geometric shapes. The first two shapes are rectangular prisms, students tell surface area and volume. The next...
Curated OER
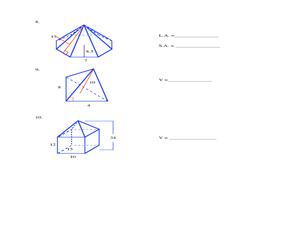
Surface Area and Volume
In this geometry worksheet, 10th graders determine the lateral area, surface area, and volume of pyramids and prisms. The one page worksheet contains five problems. Answers are not included.
Curated OER
Geometry, Measurement & Reasoning
Students measure prisms and determine their volume. In this measurement and reasoning activity, students estimate the volume and area of prisms. They measure given boxes. Students use paper to create prisms and cylinders. They determine...
Curated OER
Displacement and Density
High schoolers explore displacement. In this displacement and density lesson, pupils find the volume of irregular shaped objects. Afterward, they determine the volume of containers. They compute the amount of water displacement.
Curated OER
Geometry Worksheets-Volume of Prisms and Pyramids
In this geometry worksheet, 10th graders determine the volume of rectangular and triangular prisms and pyramids. The one page interactive worksheet contains eleven questions. Answers and hints are included.
Curated OER
Volume of Prisms
Students calculate the volume of different polygons. For this geometry lesson, students identify the relationship between base and height. They calculate the area of each prism and cylinder.
Curated OER
Volume of a Prism
In this Geometry worksheet, 10th graders determine that two prisms will have equal volumes if their bases have the same area and their altitudes are the same. The one page worksheet has one problem with the solution.
Noyce Foundation
Building Blocks
Building blocks have more uses than simply entertaining children. Young mathematicians calculate the volume of a given cube, and then calculate the volume and surface area of a prism formed from multiple cubes.
Illustrative Mathematics
Computing Volume Progression 2
Once your geometers know how to apply the formula V = l w h, they will be ready to take on the fractional volume of a fish tank. Have your number crunchers swap heights so they can see that the fractional volume will not change.