Mathematics Assessment Project
Generating Polynomials from Patterns
Patterns and polynomials go hand in hand. Budding mathematicians analyze sequences of dot diagrams to discover the patterns in the number of white dots and black dots. They use the identified patterns to write and simplify a polynomial...
CCSS Math Activities
Smarter Balanced Sample Items: High School Math – Claim 3
Communication is the key. A presentation provides 25 sample items for Claim 3, Communicating Reasoning, for the Smarter Balanced High School Math assessment. Items require pupils to answer the question and provide logical reasoning to...
Concord Consortium
From Tan to Ten
Combine simplifying trigonometric expressions with evaluating them! An open-ended question presents a trigonometric expression and numeric values for additional expressions. Learners must determine a value for the original expression by...
CK-12 Foundation
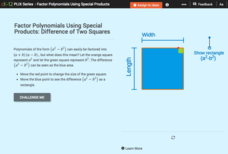
Factor Polynomials Using Special Products: Difference of Two Squares
Factoring patterns are not magic! Show your classes how to model the difference of two squares' factors using an area model. Learners manipulate the width and length of two squares to represent the polynomial a^2-b^2. They use the model...
CK-12 Foundation
Sum and Difference of Cubes: Stacking Blocks
Investigate polynomial factoring patterns by finding a connection to volume. As learners build a three-dimensional solid from smaller solids, they convert the visual model to a mathematical expression. Their models represent the sum of...
EngageNY
End-of-Module Assessment Task - Algebra 2 (Module 1)
A series of assessment tasks require learners to process information and communicate solutions. Topics include graphing parabolas, solving linear-quadratic systems, factoring polynomials, and solving polynomial equations.
EngageNY
The Power of Algebra—Finding Pythagorean Triples
The Pythagorean Theorem makes an appearance yet again in this lesson on polynomial identities. Learners prove a method for finding Pythagorean triples by applying the difference of squares identity.
EngageNY
The Power of Algebra—Finding Primes
Banks are responsible for keeping our financial information safe. Mathematics is what allows them to do just that! Pupils learn the math behind the cryptography that banks rely on. Using polynomial identities, learners reproduce the...
EngageNY
Dividing by (x – a) and (x + a)
Patterns in math emerge from seemingly random places. Learners explore the patterns for factoring the sum and differences of perfect roots. Analyzing these patterns helps young mathematicians develop the polynomial identities.
EngageNY
Putting It All Together
Shuffle 'em up and deal! Learners practice operations with polynomials using cards they pass around the room. The activity works with pairs or individuals, so it offers great flexibility. This is the fifth installment in a series of 42...
EngageNY
The Division of Polynomials
Build a true understanding of division of polynomials. Learners use their knowledge of multiplying polynomials to create an algorithm to divide polynomials. The area model of multiplication becomes the reverse tabular method of division.
EngageNY
The Multiplication of Polynomials
If you can multiply multi-digit integers, you can multiply polynomials. Learners use an area model to compare multiplying numbers to multiplying polynomials. They progress to using the distributive property.
EngageNY
Mid-Module Assessment Task - Algebra 2 (Module 1)
Challenge classes to think deeply and apply their understanding of polynomials. The assessment prompts learners to use polynomial functions to model different situations and use them to make predictions and conclusions.
EngageNY
The Special Role of Zero in Factoring
Use everything you know about quadratic equations to solve polynomial equations! Learners apply the Zero Product Property to factor and solve polynomial equations. They make a direct connection to methods they have used with quadratic...
EngageNY
Radicals and Conjugates
Make the irrational rational again! Continuing the theme from previous lessons in the series, the lesson relates the polynomial identity difference of squares to conjugates. Learners develop the idea of a conjugate through analysis and...
EngageNY
Mental Math
Faster than a speedy calculator! Show your classes how to use polynomial identities to multiply numbers quickly using mental math.
EngageNY
Comparing Methods—Long Division, Again?
Remember long division from fifth grade? Use the same algorithm to divide polynomials. Learners develop a strategy for dividing polynomials using what they remember from dividing whole numbers.
Mathematics Assessment Project
Arithmetic with Polynomials and Rational Expressions
It all starts with arithmetic. An educational resource provides four items to use in summative assessments. The items reflect the basic skill level required by the standards in the domain and are designed to have...
EngageNY
Polynomial, Rational, and Radical Relationships
This assessment pair goes way beyond simple graphing, factoring and solving polynomial equations, really forcing learners to investigate the math ideas behind the calculations. Short and to-the-point questions build on one another,...
Curated OER
Trina's Triangles
Practice evaluating polynomial expressions at the same time you find Pythagorean Triples. Look for patterns and find examples when the "formula" does and doesn't work. Finally, verify the formula by proving the identity true.
Curated OER
Area, Arithmetic and Algebra
Explore the concept of this perfect square trinomial and difference of squares lesson. A hands-on activity that uses paper rectangles and squares to illustrate the perfect-square trinomial and difference of squares formulas.
Curated OER
Finite Differences
Young mathematicians solve problems with polynomials. As they graph and analyze polynomial functions, learners define constant and finite, and determine the relationship between the constant set of differences and the slope of a linear...
Paul Dawkins
Paul's Online Notes: Algebra: Preliminaries: Polynomials
Detailed math tutorial features notes and examples that define the degree of a polynomial and illustrates how to ass, subtract, and multiply polynomials. .
Other
Quick Math: Factor Polynomials
The process of factoring is essential to the simplification of many algebraic expressions and is a useful tool in solving higher degree equations. In fact, the process of factoring is so important that very little of algebra beyond this...