Mathematics Assessment Project
Representing 3-D Objects in 2-D
How does the shape of the surface of water in a container change as water leaks out? After tackling this question, learners take part in a similar activity with more complex figures.
Mathematics Assessment Project
Sorting Equations of Circles 2
How much can you tell about a circle from its equation? This detailed lesson plan focuses on connecting equations and graphs of circles. Learners use equations to identify x- and y-intercepts, centers, and radii of circles. They also...
Mathematics Vision Project
Module 7: Modeling with Geometry
Model good modeling practices. Young mathematicians first learn about cross sections and solids of revolution. They then turn their attention to special right triangles and to the Laws of Sine and Cosine.
101 Questions
Water Tank Filling
Grab your classes' attention with a video presentation of a problem to solve. Young scholars develop a plan to predict the time it takes to fill a tank with water. Video footage provides the statistics they need to make their conclusions.
Annenberg Foundation
Geometry 3D Shapes: 3D Shapes
Explore vocabulary related to three-dimensional shapes. An instructional website describes the characteristics of different geometric solids. Learners can use an interactive component to view nets, faces, vertices, and edges of common...
101 Questions
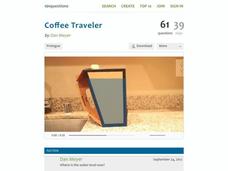
Coffee Traveler
Investigate the volume of irregular figures in an inquiry-based exercise. Presented with an irregularly shaped box filled with water, learners must predict the level of water when it is tipped on its side. The class can divide...
Shodor Education Foundation
Cross Section Flyer
Scholars see cross sections come to life using an app to investigate cross sections of three-dimensional solids. They look at prisms, pyramids, cylinders, cones, and double cones.
CPALMS
2D Rotations of Triangles
Where does the line of rotation need to be to get a cone? Pupils respond to three questions involving rotating a right triangle about different lines. The scholars describe the solid created along with providing details about its...
Teach Engineering
Discovering Relationships Between Side Length and Area
Consider the relationship between side length and area as an input-output function. Scholars create input-output tables for the area of squares to determine an equation in the first installment of a three-part unit. Ditto for the area of...
Teach Engineering
Scale Model Project
Try your hand at scale models. Scholars create a scale model of an object using a scale factor of their choice. As part of the project, they give presentations on their processes and calculations. This is the last installment of the...
Teach Engineering
Build the Biggest Box
Boxing takes on a whole new meaning! The second installment of the three-part series has groups create lidless boxes from construction paper that can hold the most rice. After testing out their constructions, they build a new box....
Noyce Foundation
Piece it Together
Score some problems all related to soccer balls. The first few problems focus on pattern blocks to see relationships between figures. More advanced problems focus on actual soccer balls, the patterns on the balls, and their volumes and...
Noyce Foundation
Cutting a Cube
Teach the ins and outs of the cube! A series of five K–12 level activities explore the make-up of the cube. The beginning lessons focus on the vocabulary related to the cube. Later lessons explore the possible nets that describe a cube....
Mathematics Assessment Project
Modeling Motion: Rolling Cups
Connect the size of a rolling cup to the size of circle it makes. Pupils view videos of cups of different sizes rolling in a circle. Using the videos and additional data, they attempt to determine a relationship between cup...
Mathematics Vision Project
Module 5: Modeling with Geometry
Solids come in many shapes and sizes. Using geometry, scholars create two-dimensional cross-sections of various three-dimensional objects. They develop the lesson further by finding the volume of solids. The module then shifts...
EngageNY
End-of-Module Assessment Task - Geometry (module 3)
It's test time! Determine your class's understanding of the topics of volume and cross sections with a thorough assessment on volume, area, and geometric shapes.
EngageNY
The Volume Formula of a Sphere
What is the relationship between a hemisphere, a cone, and a cylinder? Using Cavalieri's Principle, the class determines that the sum of the volume of a hemisphere and a cone with the same radius and height equals the volume of a...
EngageNY
Scaling Principle for Volumes
Review the principles of scaling areas and draws a comparison to scaling volumes with a third dimensional measurement. The exercises continue with what happens to the volume if the dimensions are not multiplied by the same...
EngageNY
Definition and Properties of Volume
Lead a discussion on the similarities between the properties of area and the properties of volume. Using upper and lower approximations, pupils arrive at the formula for the volume of a general cylinder.
EngageNY
General Pyramids and Cones and Their Cross-Sections
Are pyramids and cones similar in definition to prisms and cylinders? By examining the definitions, pupils determine that pyramids and cones are subsets of general cones. Working in groups, they continue to investigate the relationships...
EngageNY
Three-Dimensional Space
How do 2-D properties relate in 3-D? Lead the class in a discussion on how to draw and see relationships of lines and planes in three dimensions. The ability to see these relationships is critical to the further study of volume and...
EngageNY
How Do 3D Printers Work?
If we stack up all the cross sections of a figure, does it create the figure? Pupils make the connection between the complete set of cross sections and the solid. They then view videos in order to see how 3D printers use Cavalerie's...
EngageNY
The Volume of Prisms and Cylinders and Cavalieri’s Principle
Young mathematicians examine area of different figures with the same cross-sectional lengths and work up to volumes of 3D figures with the same cross-sectional areas. The instruction and the exercises stress that the two...
EngageNY
General Prisms and Cylinders and Their Cross-Sections
So a cylinder does not have to look like a can? By expanding upon the precise definition of a rectangular prism, the lesson develops the definition of a general cylinder. Scholars continue on to develop a graphical organizer...