Annenberg Foundation
Geometry 3D Shapes: Platonic Solids
From polyhedrons to platonic solids, here is a lesson that will have your classes talking! As an introduction to platonic solids, scholars cut and fold nets to create the three-dimensional solids. They use an interactive component to...
Texteam Geometry Institute
Geometry for the High School Classroom
What does it mean for apples to have chirality? This and other explorations in geometry, such as tiling the plane, boxing a tetrahedron, and investigating Euclid's Characteristic, are included in this resource on Euclidean geometry....
Curated OER
Poly-Mania
This hands-on lesson takes young geometers on a tour of 2D polygons and 3D polyhedrons. After exploring different web resources and discussing geometric shapes, small groups construct models of polyhedrons using bendable straws. Note:...
Curated OER
Solidly Platonic
When they do, they learn. Using this resource, young mathematicians learn about platonic solids by actually building, touching, and examining the shapes. They connect their observations about the shapes to Euler's formula.
Curated OER
Different Dice
In this different dice learning exercise, 9th graders read about the use of octahedrons as dice, review the probability with cubical dice, then answer the question of probability when rolling 2 octahedral dice.
Curated OER
Platonic Solids
A chart in this activity prompts students to list the number of faces, vertices, and edges in each of five shapes. Additionally, students provide the number of faces that meet at each vertex as well as the number of sides of each face....
University of Oregon
University of Oregon: Greek Science: Early Cosmology
Discusses the evolution of ideas about the cosmos, from the Magic Cosmology of the Neolithic age to the stage of Mythical Cosmology, to the Geometric Cosmology of the ancient Greeks. The third stage advocated a rational approach to...
![University of Waterloo: Cemc: Math Circles: 3 D Geometry Ii [Pdf] Unknown Type](https://d15y2dacu3jp90.cloudfront.net/images/attachment_defaults/resource/large/FPO-knovation.png "University of Waterloo: Cemc: Math Circles: 3 D Geometry Ii [Pdf] Unknown Type")
University of Waterloo (Canada)
University of Waterloo: Cemc: Math Circles: 3 D Geometry Ii [Pdf]
This worksheet provides a variety of 3D geometry questions requiring higher order thinking skills. Questions include; finding the surface area of a prism, surface area of pyramid, surface area of a sphere, and platonic solids.
Creative Science Centre
Creative Science Centre: Drawing the Football
This article explores the shape, symmetry and surface structure of a football (or soccer ball, depending on your nationality) to see how this understanding can help one draw these sorts of complex shapes.
Cuemath
Cuemath: 3 D Shapes
Introduces the concept of 3D shapes by learning the definition, types, properties, and three dimensions. Check-out the solved examples for better understanding and try your hand at solving a few interactive questions at the end of the page.
Cuemath
Cuemath: Platonic Solids
Learn more about platonic sold geometry, understand the platonic solid names, view some solved examples, and try your hand at some interactive problems.
Cuemath
Cuemath: Polyhedron
Introduces the concept of polyhedrons by learning the definition, types, and where they are found. Check-out the solved examples for better understanding and try your hand at solving a few interactive questions at the end of the page.
Cuemath
Cuemath: Tetrahedron
A comprehensive guide for learning all about tetrahedrons with definitions, properties, formulas, examples, and practice questions.
Dartmouth College
Dartmouth College: Mqed: Geometry in Art and Architecture
Students learn about art and mathematics. Topics include the golden ratio, platonic solids, and number symbolism. The learning resource includes a description, requirements, syllabus, and bibliography.
Wolfram Research
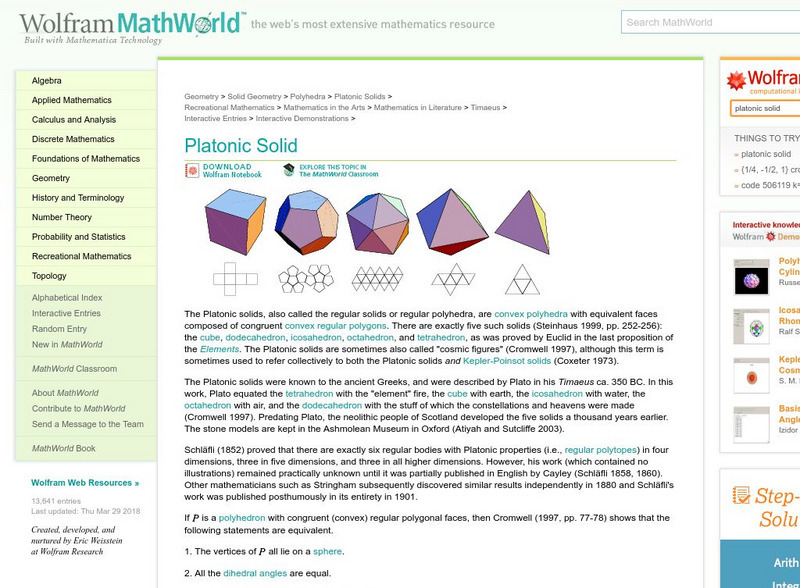
Wolfram Math World: Platonic Solids
This site, which is provided for by Math World, is a lengthy site describing the various properties and mathematical explanations of the figures.
Shodor Education Foundation
Shodor Interactivate: Lesson: Probability and Geometry
The Shodor Education Foundation provides this lesson plan to give students an opportunity to connect probabilty and geometry. Students learn how to calculate probability, use geometry to solve probabilty problems, and, as an added bonus,...
Maths Challenge
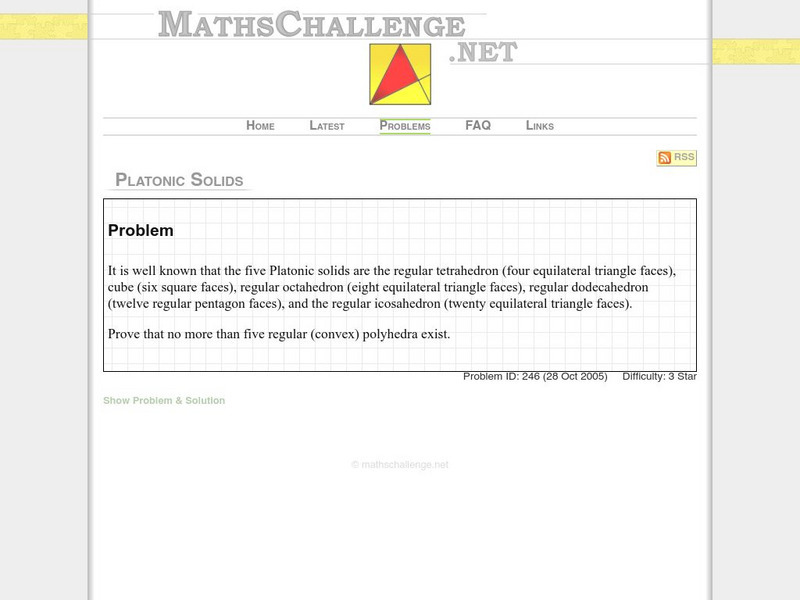
Maths challenge.net: Platonic Solids
A "three star geometry problem" requiring knowledge of how to complete geometric proofs to prove that there are no more than five regular convex polyhedra.
University of Texas at Austin
University of Texas: Elementary Math Club: Math Mobile
Learners explore the geometric and topological properties of three-dimensional objects. The lesson plan includes an overview and a activity toolkit.
Annenberg Foundation
Annenberg Learner: Geometry 3 D Shapes: Platonic Solids
Learn about platonic solids, a special type of polyhedra. Read a definition, make three-dimensional models using printouts, and play with an interactive animation, which will show you the faces, vertices, and edges on platonic solids:...
Math Is Fun
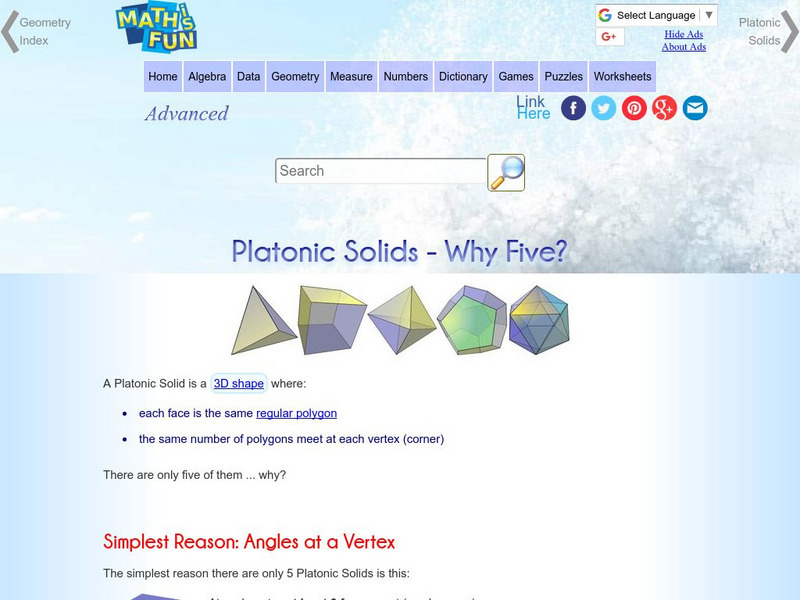
Math Is Fun: Platonic Solids: Why Five?
Use Euler's Formula and properties of known platonic solids' faces, edges, and vertices to determine why only five platonic solids exist.
University of Georgia
University of Georgia: Inter Math: Platonic Solids
This site gives a description of platonic solids along with a visual representation of each of the five platonic solids. At the bottom of the page are links to cut-out-templates for each of the platonic solids. Be sure to follow the...
Walter Fendt
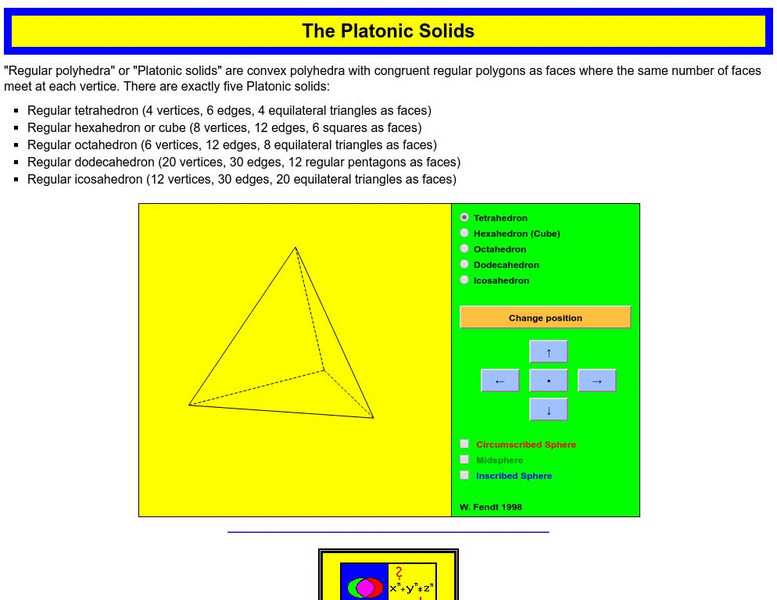
Walter Fendt: The Platonic Solids
A short interactive activity to visualize the different platonic solids. You can also change the axis of rotation.
Mathigon
Mathigon: Mathematical Treasure Hunt
The mathematical treasure hunt allows students to follow a trail of clues and mathematical problems around the school site; each clue contains a hint to where the next clue is hidden.
Creative Science Centre
Creative Science Centre: Shapes Platonic and Archimedean Solids
The 'Platonic solids' are three-dimensional shapes formed by putting together identical regular faces. Every corner and every edge is identical to every other corner and edge. There are five such 'solids': the tetrahedron, cube,...