Concord Consortium
From Tan to Ten
Combine simplifying trigonometric expressions with evaluating them! An open-ended question presents a trigonometric expression and numeric values for additional expressions. Learners must determine a value for the original expression by...
Concord Consortium
Broken Spreadsheet II
Work in reverse with the product becoming the given. Using a spreadsheet image of the graph of a trigonometric function, young scholars investigate methods of creating spreadsheet data that results in the given graph. The catch? The data...
Concord Consortium
Here Comes the Sun
Many phenomena in life are periodic in nature. A task-based lesson asks scholars to explore one of these phenomena. They collect data showing the sunrise time of a specific location over the period of a year. Using the data, they create...
Concord Consortium
Transformations Resource
Transform your lesson for transforming functions. Scholars transform linear, quadratic, exponential, rational, and trigonometric expressions. They write their expressions to fit specific forms and identify the values of the resulting...
Concord Consortium
Adding the Sines
It's a sign! Scholars analyze sine functions for patterns in their periods. The exploration advances beyond a simple function to the combination of two functions with even and odd coefficients. Their goal is to find a pattern between the...
CCSS Math Activities
Ferris Wheel
Wheel the resource right into your classroom. Young mathematicians use given dimensions of a Ferris wheel to write a height versus time function. They use their functions to answer a set of questions about the Ferris wheel.
Concord Consortium
Betweenness V
Take a unique approach to study the graphing of trigonometric functions. Young scholars consider two sine functions and write three functions that will lie between the two given. They use a graphing utility to assist in their explorations.
Project Maths
Trigonometric Functions
From a circle to a cycle! The final lesson of a five-part series challenges learners to use points from the unit circle to plot a repeating pattern. The repeating patterns become the graphs of the trigonometric functions. Scholars...
101 Questions
Ferris Wheel
Around and around you'll go! Learners analyze the periodic nature of a Ferris wheel. Using a trigonometric function, they make predictions about the location of a specific car at the end of the ride and its total trips around the circle.
National Council of Teachers of Mathematics
Tidal Waves
Periodically ship the class a trigonometric application. Pupils model the level of water in a port. Using their models, learners determine the times that a ship can safely navigate into and out of the port, along with determining other...
Mathematics Assessment Project
Representing Functions of Everyday Situations
Functions help make the world make more sense. Individuals model real-world situations with functions. They match a variety of contexts to different function types to finish a helpful resource.
Mathematics Assessment Project
Representing Trigonometric Functions
Discover the classic example of periodicity: Ferris wheels. Young mathematicians learn about trigonometric functions through Ferris wheels. They match functions to their graphs and relate the functions to the context.
CK-12 Foundation
Derivatives of Trigonometric Functions: Derivative of sin(x)
Graphically find the derivative of sin(x). Using the interactive, pupils graph the slope of the tangent line to the sine function. Class members use the resulting graph to determine the derivative of the sine function. They verify their...
CK-12 Foundation
Basic Trigonometric Limits: Evaluating Limits of tan(x)
Chase a periodic moving limit. Learners graphically determine the limit of the tangent function at different input values. Using sliders, pupils find out whether the tangent function approaches the same value from the left and the right....
GeoGebra
Getting on the Right Wavelength
Predict an equation that waves up and down. Pupils set the height, radius, and period of a Ferris wheel. The learners write a sine equation to match the graph of the height of a point on the wheel as a function of time. Running the...
GeoGebra
More Ferris Wheels
Take a ride on a Ferris wheel. Using sliders to adjust the parameters of a Ferris wheel, pupils investigate the height of a point over time. The interactive traces out the curve on a time-height graph. Learners use what they learned to...
CK-12 Foundation
Vertical Translations: Vertical Shift of Sinusoidal Functions
Create a shift in TV viewing habits. The interactive presents a cosine model of an individual's TV viewing habits during a year. Class members move the model to reflect given conditions. Finally, they determine key features from the...
CK-12 Foundation
Unit Circle: Medieval Castle Defense
Who needs a plan — let trigonometry protect you! Pupils determine the angle of an approaching enemy to a village wall. The scholars determine the exact value of trigonometric functions for the angle. Class members use trigonometry to...
CK-12 Foundation
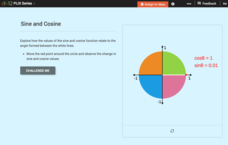
Domain, Range, and Signs of Trigonometric Functions: Sine and Cosine
Is there a relationship between the sign of sine and cosine and the angle on the unit circle? Scholars use an interactive to see the value of sine and cosine within different quadrants. they then use the information to determine the...
CK-12 Foundation
Reciprocal Identities
Get a better understanding of the trigonometric functions by taking a look at the flip side. Pupils create angles within a unit circle and compare the values of the standard trigonometric functions to their reciprocal functions. The...
CK-12 Foundation
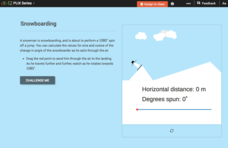
Trigonometric Functions of Angles Greater than 360 Degrees: Snowboarding
Spin through the trigonometric functions. Scholars determine the angle of rotation a snowboarding snowman makes at various distances in his jump. The class members then calculate the values of trigonometric functions for those angles.
CK-12 Foundation
Horizontal Translations or Phase Shifts: Cosine
If cosine is shifted, how is its equation affected? Learners manipulate the graph of cosine by moving the y-intercept to different locations on the coordinate plane. Pupils determine the new equation that models the shifts.
CK-12 Foundation
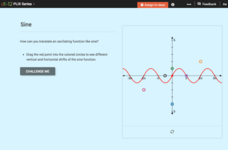
Horizontal Translations or Phase Shifts: Sine
Shift a trigonometric function and find its new equation. Pupils translate a sine function on a graph. The scholars determine the equation of the function that represents the translated graph and observe the connection between a...
CK-12 Foundation
Horizontal Translations or Phase Shifts: Tangent
Patterns can be shifty! Find the pattern when shifting the graph of tangent. Pupils move the graph of tangent to different locations on the coordinate plane. They observe what happens to the function and its vertical asymptotes...