Math Medics
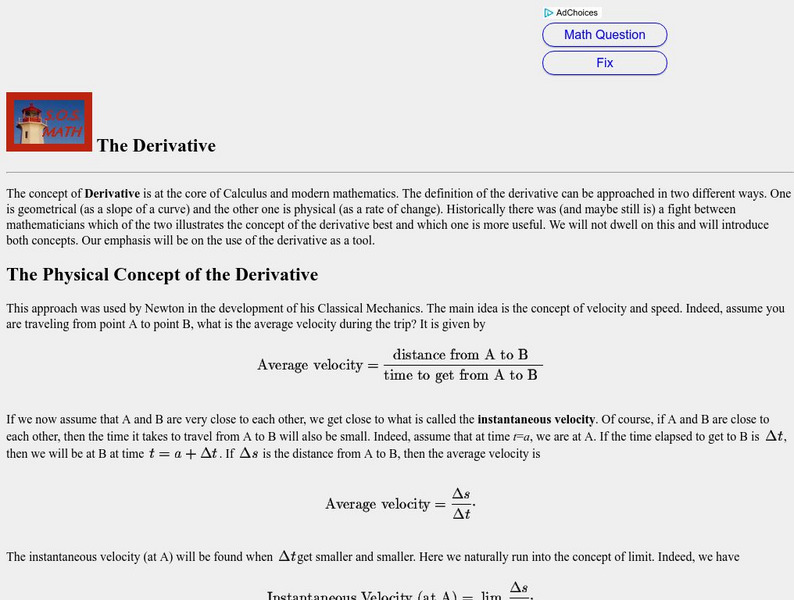
S.o.s. Math: The Derivative
This lesson does a nice job of clarifying what it means to find a derivative. The lesson gives an algebraic method for finding the derivative using limits and offers a geometric interpretation of the derivative.
Dartmouth College
Dartmouth College: Mqed: Rates of Change
The resource investigates rates of change using the program STELLA. Discussion questions and exercises are included in the monograph.
Texas Instruments
Texas Instruments: Balloon
Students inflate a balloon and observe the relationship between the rate its volume is changing and the rate points on its surface are getting closer to each other. They use the their calculator to determine the exact rate of change.
Texas Instruments
Texas Instruments: Rate of Change
This activity is designed for students who have already learned the definition of the derivative and that it can be used to determine the slope of a curve. (Continued. See "before the activity.")
Ministry of Education and Universities of the Region of Murcia (Spain)
Ministerio De Educacion Y Ciencia: Interpretacion Geometrica De La Derivada
In Spanish. This interactive unit seeks to familiarize you with the concepts of secant and tangent to a curve. You will be able to take a look at how the approximation is produced and understand the limit as a process that you can see...
Texas Instruments
Texas Instruments: Move My Way a Cbr Analysis of Rates of Change
In this activity, students use the motion detector to collect position data and study the relationship between position and velocity. They explore the relationship between functions and their derivatives. Students learn to connect...
CK-12 Foundation
Ck 12: Analysis: Instantaneous Rates of Change
[Free Registration/Login may be required to access all resource tools.] In this lesson students learn the difference between average rate of change and instantaneous rate of change. Students examine guided notes, review guided practice,...
Interactive Mathematics
Interactive Mathematics: Instantaneous Rate of Change
The derivative is applied to real world applications of velocity in an example using "h" notation and limits.