Virginia Department of Education
How Many Triangles?
Something for young mathematicians to remember: the sum of any two sides must be greater than the third. Class members investigates the Triangle Inequality Theorem to find the relationship between the sides of a triangle. At the same...
EngageNY
Rotations
Searching for a detailed lesson to assist in describing rotations while keeping the class attentive? Individuals manipulate rotations in this application-based lesson depending on each parameter. They construct models depending on the...
PBS
Accessible Shapes
All the 2-D and 3-D measurement work you need is in one location. Divided into three sections, the geometry lesson plans consist of visualization of three dimensions, classifying geometric figures, and finding surface area and volume....
Concord Consortium
Triangles: Angle Space
Three angles in a triangle, three-dimensional space, it all seems connected somehow. Given several different triangles, pupils use the three angles of the triangle as coordinates to plot points in three-dimensional space. They explore...
Curated OER
Applying Special Right Triangles
In this geometry worksheet, 10th graders use the theorems regarding right triangles to find the missing length in a special right triangle. The one page worksheet contains eleven questions. Answers are included.
EngageNY
Secant Lines; Secant Lines That Meet Inside a Circle
Young mathematicians identify different cases of intersecting secant lines. They then investigate the case where secant lines meet inside a circle.
Noyce Foundation
Parallelogram
Parallelograms are pairs of triangles all the way around. Pupils measure to determine the area and perimeter of a parallelogram. They then find the area of the tirangles formed by drawing a diagonal of the parallelogram and compare their...
Curated OER
Why Does ASA Work?
Your geometry learners explore Angle-Side-Angle congruence in this collaborative task. The sum of the interior angles of all triangles being one hundred eighty degrees, is the key learners will discover as they explain their reasoning...
University of Utah
Geometry: Angles, Triangles, and Distance
The Pythagorean Theorem is a staple of middle school geometry. Scholars first investigate angle relationships, both in triangles and in parallel lines with a transversal, before proving and applying the Pythagorean Theorem.
Illustrative Mathematics
Calculations with Sine and Cosine
Practice makes perfect and perfecting those trigonometric functions are vital in trigonometry. The task requires evaluating cosine and sine values at common degree measures before looking at the results. Is there a pattern when the...
It's About Time
Reflected Light
The lesson allows young scientists to use lasers and mirrors to study reflected light. A reading passage and homework question assess learning, while additional material introduces extension activities.
EngageNY
Arc Length and Areas of Sectors
How do you find arc lengths and areas of sectors of circles? Young mathematicians investigate the relationship between the radius, central angle, and length of intercepted arc. They then learn how to determine the area of sectors of...
Willow Tree
Circle Graphs
Pie isn't just for eating! Scholars learn to create pie charts and circle graphs to represent data. Given raw data, learners determine the percent of the whole for each category and then figure out the degree of the circle that percent...
Alabama Learning Exchange
Triangle Congruence with Rigid Motion
Combine transformations and triangle congruence in a single lesson. Scholars learn to view congruent triangles as a rigid transformation. Using triangle congruence criteria, learners identify congruent triangles and the rigid...
Bowland
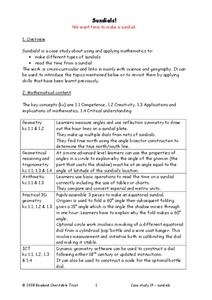
Sundials!
Time to learn about sundials. Scholars see how to build sundials after learning about Earth's rotation and its relation to time. The unit describes several different types of possible sundials, so choose the one that fits your needs — or...
EngageNY
Congruence Criteria for Triangles—ASA and SSS
How do you know if a pair of triangles are congruent? Use the lesson to help class members become comfortable identifying the congruence criteria. They begin with an exploration of ASA and SSS criteria through transformations and...
CCSS Math Activities
Smarter Balanced Sample Items: 7th Grade Math – Target F
Sometimes it's how you ask the question that counts. The sixth installment of nine from the Smarter Balanced Claim 1 Slideshow series presents a set of 13 questions to assess understanding of angle relationships as well as area and...
EngageNY
Reflections
Facilitate creativity in your math class as individuals learn the definition of a geometric reflection and correctly construct a model, as well as its reflected image. They use a perpendicular bisector and circles to elaborate on...
Curated OER
Informal Geometry
Middle and high schoolers solve and complete 29 various types of problems. First, they match each name with its correct formula. Then, students find the length of the missing side and the distance between the two points given. In...
Bowland
Rods and Triangles
Scholars explore triangles with rods of different lengths. Using rods of 2, 4, 6, 8, and 10 cm class members build as many different types of triangles as they can. They also describe properties of these triangles and determine...
Bowland
Three of a Kind
One is chance, two is a coincidence, three's a pattern. Scholars must determine similarities and differences of a regular hexagon undergoing dilation. They look at lengths, angles, areas, and symmetry.
K12 Reader
Punctuating Appositives
Where do the commas go? Kids rewrite a series of sentences using commas to set off the appositives in each sentence.
Noyce Foundation
Which is Bigger?
To take the longest path, go around—or was that go over? Class members measure scale drawings of a cylindrical vase to find the height and diameter. They calculate the actual height and circumference and determine which is larger.
Jefferson Lab
Optics: Mirrors and Lenses
Did you see that or did I imagine it? Optical illusions are often created with mirrors and lenses, and here is a presentation that covers many different types of mirrors and lenses and how they work. Flat, concave, and convex mirrors, as...
Other popular searches
- Measuring Classifying Angles
- Measuring Interior Angles
- Measuring and Drawing Angles
- Measuring Reflex Angles
- Measuring Triangle Angles
- Measuring Central Angles
- Measuring Inscribed Angles
- Measuring Polygons Angles
- Measuring Segments and Angles