EngageNY
Distance and Complex Numbers 1
To work through the complexity of coordinate geometry pupils make the connection between the coordinate plane and the complex plane as they plot complex numbers in the 11th part of a series of 32. Making the connection between the two...
EngageNY
Matrix Notation Encompasses New Transformations!
Class members make a real connection to matrices in the 25th part of a series of 32 by looking at the identity matrix and making the connection to the multiplicative identity in the real numbers. Pupils explore different matrices and...
EngageNY
Solving Equations Involving Linear Transformations of the Coordinate Space
Explore methods for solving linear systems with your classes and introduce learners to using matrices as a viable method. Scholars are able to recognize situations where matrices are the efficient method of solving. Application questions...
EngageNY
Using Expected Values to Compare Strategies
Discover how mathematics can be useful in comparing strategies. Scholars develop probability distributions for situations and calculate expected value. They use their results to identify the best strategy for the situation.
EngageNY
Modeling with Inverse Trigonometric Functions 2
Use inverse trigonometric functions to work with ramps, rabbits, and Talladega. The class models real-world situations with trigonometric functions and solves them using inverses in the 15th installment of a 16-part series. Pupils solve...
EngageNY
Putting the Law of Cosines and the Law of Sines to Use
Use the Law of Cosines and the Law of Sines to solve problems using the sums of vectors. Pupils work on several different types of real-world problems that can be modeled using triangles with three known measurements. In the process,...
EngageNY
Solving Equations Involving Linear Transformations of the Coordinate Plane
How can matrices help us solve linear systems? Learners explore this question as they apply their understanding of transformation matrices to linear systems. They discover the inverse matrix and use it to solve the matrix equation...
EngageNY
Matrix Addition Is Commutative
Explore properties of addition as they relate to matrices. Using graphical representations of vector matrices, scholars test the commutative and associative properties of addition. They determine if the properties are consistent for...
Curated OER
The Football and Braking Distance: Model Data with Quadratic Functions
Students engage in a lesson plan that is about the concept of data analysis with the use of quadratics. They use a Precalculus text in order to give guidance for independent practice and to serve as a source for the teacher to use. The...
EngageNY
Estimating Probability Distributions Empirically 2
Develop probability distributions from simulations. Young mathematicians use simulations to collect data. They use the data to draw graphs of probability distributions for the random variable in question.
EngageNY
Inverses of Logarithmic and Exponential Functions
Revisit the relationship between logarithms and exponentials. Learners review the notion of logarithms as the way to solve exponential equations in the 21st segment in a Pre-calculus series of 23. Pupils use the knowledge to prove that...
EngageNY
The Binomial Theorem
Investigate patterns in the binomial theorem. Pupils begin by reviewing the coefficients from Pascal's triangle. They look at the individual terms, the sums of the coefficients on a row, and the alternating sum of each row. Individuals...
EngageNY
Volume and Cavalieri’s Principle
Take a slice out of life. The ninth section in a series of 23 introduces classmates to Cavalieri's principle using cross sections of a cone and stacks of coins. Class members participate in a discussion using pyramids and how Cavalieri's...
EngageNY
End Behavior of Rational Functions
Connect end behavior to previous learning. Pupils connect finding the end behavior of rational functions to finding end behavior of polynomial functions. The 13th segment in a 23-part unit starts with finding the end behavior or power...
EngageNY
Rational Functions
Make a connection between rational expressions and rational functions. Pupils review simplifying and performing operations on rational expressions and recall what it means for two rational expressions to be equivalent based on their...
EngageNY
Restricting the Domain
But what if the function cannot be inverted? Pupils continue to work with inverses of functions using tables, graphs, and algebraic equations. They restrict the domain of non-invertible functions to make them invertible. Using...
EngageNY
Solving Problems by Function Composition
Stay composed while solving problems. Learners put their knowledge of compositions to solve problems. To connect with the concept, scholars compose equations to answer questions from real-world situations. Finally, pupils practice using...
Curated OER
Law of Sines and Law of Cosines
For this Pre-calculus/Trigonometry worksheet, students use the laws of sines and cosines to solve triangles. The four page worksheet contains thirteen problems. Answers are not included.
Curated OER
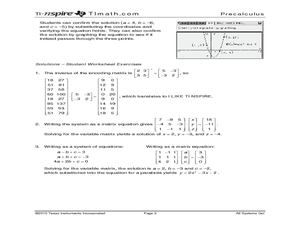
All Systems Go!
Secret codes are so much fun, and a great way to practice nearly any math skill. Let your class become code breakers as they investigate inverse matrices. They use TI-Nspire technology to solve systems of equations which help them crack...
Curated OER
Going Back to Your Roots
Investigate the Fundamental Theorem of Algebra and explore polynomial equations to determine the number of factors, the number of roots, and investigate multiplicity of roots.
EngageNY
Polynomial, Rational, and Radical Relationships
This assessment pair goes way beyond simple graphing, factoring and solving polynomial equations, really forcing learners to investigate the math ideas behind the calculations. Short and to-the-point questions build on one another,...
Alabama Learning Exchange
Logarithms: Undo the Exponential
Rumor has it that an exponential can be undone. After playing a rumor game to model exponential growth, pupils learn about undoing exponential functions. They use the definition of the logarithm to convert exponential equations to...
Alabama Learning Exchange
Triangle Area: No Height? Use the Sine
No height? No problem! Learners use their knowledge and a little help from GeoGebra to develop the Law of Sines formula. The Law of Sines helps to determine the height of triangles to calculate the area.
Curated OER
NUMB3RS Activity: Chains and Pyramids
Watch an episode of the TV show, NUMB3RS and then explore the mathematics of chain letters and pyramid schemes, both of which involve geometric progressions and exponential growth. They discuss why both are dangerous and illegal.