Multiplying Decimals Lessons
By using visual aids, and explaining mathematical language students can get a concrete grasp of how to multiply decimals.
By Christina Lee
When teaching students how to multiply decimals, I focus on two instructional strategies - the first is to focus on language, in particular the use of the word "of", and second is the use of grids to visually represent a multiplication sentence.
The word "of" is important to understand in this context because it is used when multiplying decimals with a grid. For example, 0.5 x 2 = 1, this can be understood as 0.5 or 1/2 of 2 is 1. Without attempting to actually solve the multiplication sentence, and relying on prior knowledge, most students should be able to understand this example. The understanding of the word "of" is a useful tool for teaching students how to multiply decimals. In essence, multiplying decimals is really taking a part of a whole or a part of another part.
Grids are another excellent tool. It allows students to visualize how a product is achieved when multiplying decimals. Students understand that 8 x 5 = 40, and by multiplying two numbers together the product is a bigger number. With multiplication, students are under the impression that numbers simply multiply or get bigger. However, 0.8 x 0.5 = .4 is a difficult concept for students to understand because multiplying 0.8 and 0.5 results in what appears to be a "smaller" number. Also, a good understanding of why one must count decimal places is needed and grids are an excellent tool to visualize this idea. By seeing a visual representation on a grid, students are able to conceptualize an abstract concept.
Using a 10 x 10 grid, a review of decimals on a grid should take place first. I model 0.8 for students by shading in 8 of the 10 rows on the grid. In addition, a few examples (such as 0.01, 0.35 and 0.99) can be used so that students understand the value of each square and row. Once an understanding of shading decimals is established, I model a multiplication sentence with decimals.
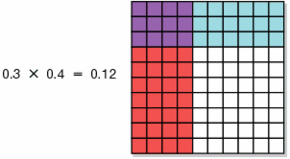
You could use this sentence 0.3 x 0.4 = 0.12 as an example. First I shade (with one color) 0.4, four rows or forty squares. After doing so, I shade (with a different color) three tenths of 0.4, or three rows of 0.4. The squares which have both colors show the product 0.12, or 12 squares. The activities and lessons below are great resources to build on the lesson shared above.
Multiplying Decimals Activities:
This worksheet provides practice with decimals. Students need to determine the decimal based on what part of the hundreds grid is shaded. This worksheet could also be used to multiply decimals.
This worksheet has additional practice with determining the decimal based on the shaded and unshaded portion of the grids.
This is a challenging enrichment activity. Students multiply two decimals, have to find the missing section that completes the numbered grid, and then solve the multiplication problem.