Mathigon
Mathigon: Geometry: Triangles and Trigonometry: Pythagoras' Theorem
Pythagoras' Theorem: In any right-angled triangle, the square of the length of the hypotenuse (the side that lies opposite the right angle) is equal to the sum of the squares of the other two sides. (A+B=C)
Illustrative Mathematics
Illustrative Mathematics: G Srt Shortest Line Segment From a Point P to a Line L
In this task, students develop an understanding that the shortest path from a point to a line always meets the line at a right angle. Aligns with G-SRT.C.8.
Illustrative Mathematics
Illustrative Mathematics: G Srt Pythagorean Theorem
The purpose of this task is to prove the Pythagorean Theorem using similar triangles. Aligns with G-SRT.B.4.
Illustrative Mathematics
Illustrative Mathematics: G Srt, G Mg How Far Is the Horizon?
Some friends are at the beach looking out onto the ocean on a clear day and they wonder how far away the horizon is. A second problem asks how far the horizon would be from the top of a mountain. Students must also answer a question...
Illustrative Mathematics
Illustrative Mathematics: G Srt Ask the Pilot
In the July 2013 issue of United Airlines' Hemisphere Magazine, a pilot responded to a question about how far in the distance he could see at different altitudes. The solution involved right triangles, lines of tangency to a circle, and...
Illustrative Mathematics
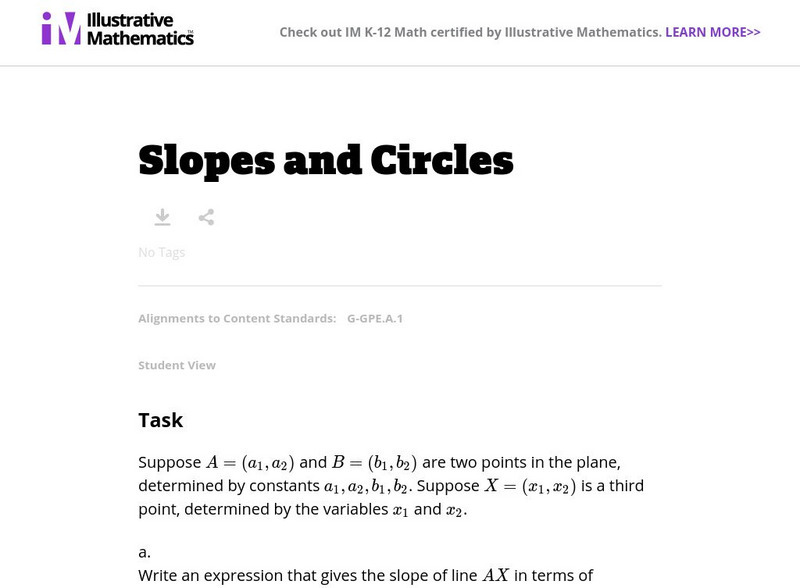
Illustrative Mathematics: G Gpe Slopes and Circles
The purpose of this task is to lead students through an algebraic approach to a well-known result from classical geometry, namely, that a point X is on the circle of diameter AB whenever angle AXB is a right angle. This consists of...
Illustrative Mathematics
Illustrative Mathematics: G Gpe Explaining the Equation for a Circle
This problem examines equations defining different circles in the x-y plane. Students must use the Pythagorean Theorem to find equations whose solutions are points on the circle and explain why they work. Includes a GeoGebra worksheet...
Illustrative Mathematics
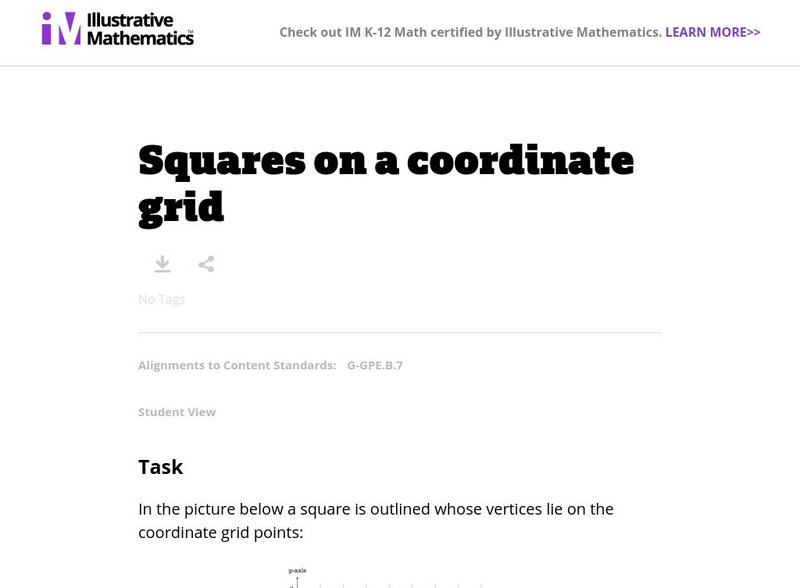
Illustrative Mathematics: G Gpe Squares on a Coordinate Grid
The purpose of this task is to use the Pythagorean Theorem and knowledge about quadrilaterals in order to construct squares of different sizes on a coordinate grid. Aligns with G-GPE.B.7.
Illustrative Mathematics
Illustrative Mathematics: G Gpe Defining Parabolas Geometrically
The purpose of this task is to show that the points on a parabola can be characterized by being equidistant from a point (the focus) and a line (the directrix). Aligns with HSG-GPE.A.2.
Illustrative Mathematics
Illustrative Mathematics: G Gpe Triangle Perimeters
Students are shown two triangles touching points on a coordinate grid and are asked to answer questions about their perimeters. The purpose of this task is to apply the Pythagorean theorem to calculate distances and areas. Aligns with...
Illustrative Mathematics
Illustrative Mathematics: F Tf Special Triangles 1
Using known facts about the unit circle and isosceles triangles together with the Pythagorean Theorem, students can derive the sine and cosine of special angles. Aligns with HSF-TF.A.3.
Illustrative Mathematics
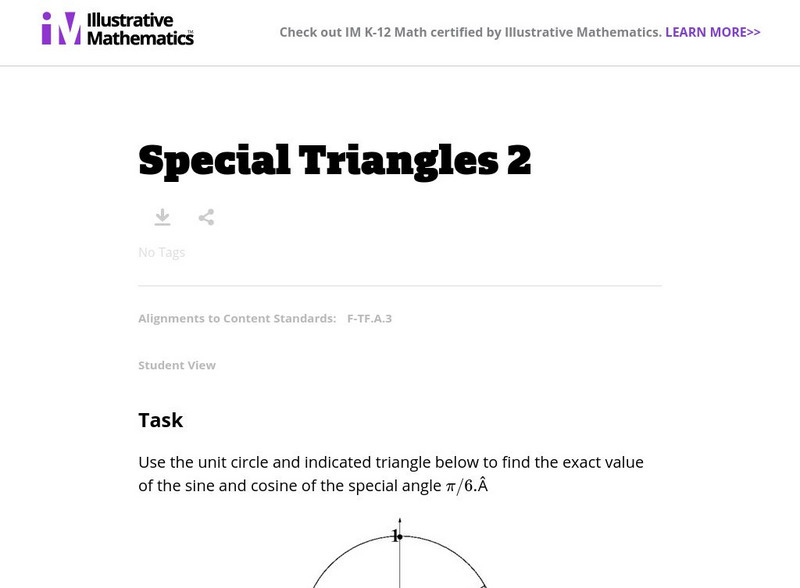
Illustrative Mathematics: F Tf Special Triangles 2
Using known facts about the unit circle and isosceles triangles together with the Pythagorean Theorem, students can derive the sine and cosine of special angles. Aligns with HSF-TF.A.3.
Other
Mathway: Algebra Formulas
Comprehensive resource provides algebra formulas for exponents, absolute value, factoring Pythagorean Theorem, linear equations, quadratic formula, distance formula, midpoint formula, and slope of a line through two points.
TED Talks
Ted: Ted Ed: How Many Ways Are There to Prove the Pythagorean Theorem?
Betty Fei details these three famous Pythagorean theorem proofs.
Illustrative Mathematics
Illustrative Mathematics: 8.g.b.7: Spiderbox
The purpose of this task is for students to work on their visualization skills and to apply the Pythagorean Theorem. Aligns with 8.G.B.7.
Illustrative Mathematics
Illustrative Mathematics: 8.g a Rectangle in the Coordinate Plane
In this task, 8th graders are shown a picture of a quadrilateral on a coordinate grid and are asked to use the Pythagorean Theorem to find lengths and to explain why the quadrilateral is a rectangle. Aligns with 8.G.B.
Illustrative Mathematics
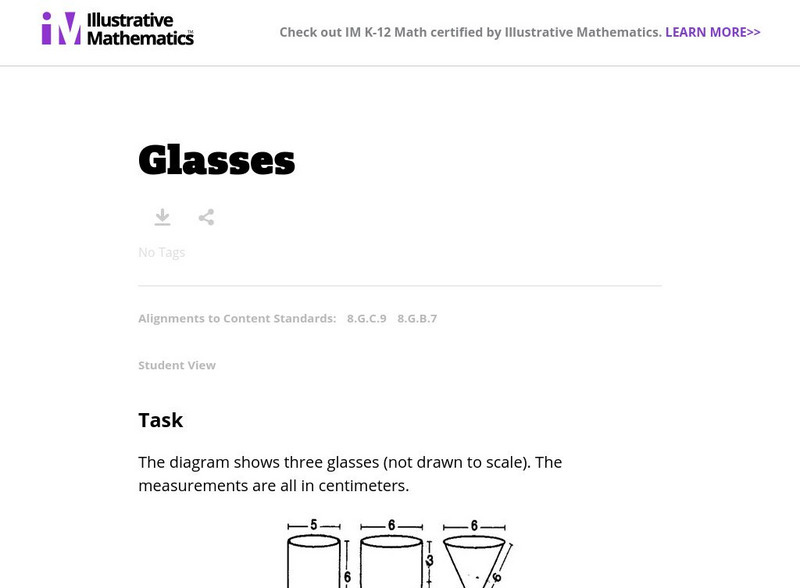
Illustrative Mathematics: 8.g Glasses
In this task, 8th graders are presented with a drawing of three glasses of different shapes and their measurements. They are asked to make several calculations that require the application of the Pythagorean Theorem and volume formulas....
Illustrative Mathematics
Illustrative Mathematics: 8.g, G Srt Points From Directions
For this problem, students must carefully read the description of a triangle and a fourth point that lies outside it and draw a picture to represent the problem. They must then use the Pythagorean Theorem to find side lengths. Aligns...
Illustrative Mathematics
Illustrative Mathematics: 8.g Area of a Trapezoid
Eighth graders are given various dimensions of a trapezoid and are asked to find its area. This requires them to use the Pythagorean Theorem and to decompose the trapezoid into other polygons. Aligns with 8.G.B.7.
Illustrative Mathematics
Illustrative Mathematics: 8.g Two Triangles' Area
In this task, 8th graders are given the dimensions of two isosceles triangles and are asked to compare their areas. They must draw them and use the Pythagorean Theorem and the triangle area formula to solve the problem. Aligns with 8.G.B.7.
Illustrative Mathematics
Illustrative Mathematics: 8.g Finding Isosceles Triangles
This task asks students to examine three triangles in the coordinate plane and explain why they are isosceles triangles. Aligns with 8.G.B.8.
Illustrative Mathematics
Illustrative Mathematics: 8.g Converse of the Pythagorean Theorem
This task asks students to explain what the Pythagorean Theorem and its converse are, how the converse works, and why it is true. Aligns with 8.G.B.6.
Illustrative Mathematics
Illustrative Mathematics: 8.g Bird and Dog Race
In this race between a bird and a dog, 8th graders are provided with information about their starting locations on a map and their speeds. They are asked to predict a winner, draw the paths they might follow, decide how to determine who...
Illustrative Mathematics
Illustrative Mathematics: 8.g.7 Running on the Football Field
This task asks students to apply the Pythagorean Theorem to find the approximate number of yards two players ran during a football game and compare their distances. Aligns with 8.G.B.7.