College Board
2005 AP® Calculus AB Free-Response Questions
Develop an awareness of the exam. Pupils and teachers use the six release free-response questions to see how the exam covers topics. The questions are divided among calculator and non-calculator items. Scholars see the importance of...
College Board
2003 AP® Calculus AB Free-Response Questions
Take a free look. Released free-response questions from the 2003 AP® Calculus AB exam show how questions cover different topics. Pupils and teachers review the topics of bounded regions, particle movement, rates, relationships between...
College Board
2002 AP® Calculus AB Free-Response Questions
Peek behind the curtain. Using a helpful resource, teachers and pupils see the way concepts appear on the AP® Calculus exam. Scholars use the questions to prepare for their own exams, gaining quality practice before test day. Questions...
College Board
1999 AP® Calculus AB Free-Response Questions
Gain an understanding of the test before taking your own. Scholars develop an understanding of how calculus concepts appear on the AP® exam. The six released questions come from across the curriculum and include particle movement and...
College Board
2004 AP® Calculus AB Free-Response Questions
Develop a deeper understanding of calculus. The released item and support materials allows teachers and pupils to dig deeper into calculus topics. Scholars use the commentary and descriptions of the items to gain a better understanding...
College Board
2001 AP® Calculus AB Free-Response Questions
Take a closer look at the exam. Released items from the 2001 Calculus AB exam allow teachers and pupils to view how the exam presents questions. Scholars use the questions to prepare for the test and compare their responses to the...
College Board
1998 AP® Calculus AB Free-Response Questions
Take a peek into the exam. Released items from the Calculus AB exam provide pupils insight into how questions appear on the exam. The six questions range from finding the area under a curve and the volume of the revolution to working...
Corbett Maths
Area under a Graph
What? The calculation of area is a linear distance? A short video shows how to use the areas of simple polygons to estimate the area under a graph. Pupils divide the area under a curve into figures to easier calculate the area. Given...
Project Maths
Integral Calculus
From derivatives to antiderivatives and back again. Building on the second lesson of the three-part series covering functions, learners explore the concept of an antiderivative. They connect the concept to the graph of the function and...
Shodor Education Foundation
InteGreat!
Take a great look at integration! Young scholars use an interactive to learn how to integrate a function. Learners input a function and view the integral as well as see a graphical representation.
Shodor Education Foundation
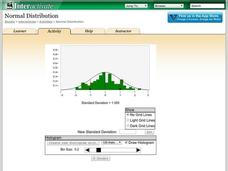
Normal Distribution
Does the size of the bin matter? The resource allows pupils to explore the relationship between the normal curve and histograms. Learners view histograms compared to a normal curve with a set standard deviation. Using the interactive,...
Shodor Education Foundation
Overlapping Gaussians
Adjust the overlap to compare probabilities. Using sliders, learners adjust the shape of two Gaussian curves. The interactive calculates the area of the left tail for one curve and the right tail for the other. Pupils set the interactive...
CK-12 Foundation
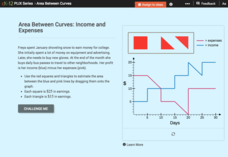
Area Between Curves: Income and Expenses
Use the area of polygons to calculate the area between curves. Pupils calculate areas under income and expense curves by filling the space with squares and right triangles. Using that information, they determine the profit related to the...
CK-12 Foundation
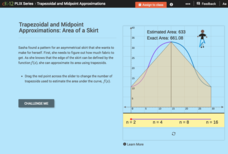
Trapezoidal and Midpoint Approximations: Area of a Skirt
When are trapezoids better than rectangles? Using trapezoids pupils approximate the area of fabric defined by a function. Just like with rectangles, learners realize the more trapezoids the more accurate the approximation. Scholars use...
CK-12 Foundation
Properties of Definite Integrals
Close your eyes and visualize a definite integral. An interactive provides a visualization of a function and definite integral on a closed interval. Pupils move one point of the interval in either the positive or negative direction....
CK-12 Foundation
Area Sums: Estimation with Rectangles
The more rectangles, the better the estimate. Using the interactive, pupils explore estimating the area under a curve using left-hand sums. Learners respond to challenge questions on how to get better estimates using the same technique.
CK-12 Foundation
Properties of a Normal Distribution: Uniform Distributions
There's nothing normal about an extraordinary resource. Scholars change the dimensions of a normal distribution using a slider interactive. Determining the area under the graph gives probabilities for different situations.
EngageNY
Normal Distributions (part 2)
From z-scores to probability. Learners put together the concepts from the previous lessons to determine the probability of a given range of outcomes. They make predictions and interpret them in the context of the problem.
Teach Engineering
Exploring Acceleration with an Android
Small groups use rubber bands to accelerate an Android device along a track of books. They collect the acceleration data and analyze it in order to determine the device's velocity.
Houston Area Calculus Teachers
Area and Volume
An AP calculus lesson begins with an activity to determine the volume of a solid using a cross-section. Next, learners review the ways to find the area between two curves , as well how to find the volume of a solid created...
Shodor Education Foundation
InteGreat
Hands-on investigation of Riemann sums becomes possible without intensive arithmetic gymnastics with this interactive lesson plan. Learners manipulate online graphing tools to develop and test theories about right, left, and...
Curated OER
Area Under A Curve
Calculus students use the derivative and integral to solve problems involving areas. They calculate the area under a curve as they follow a robot off road making different curves along the drive, using Riemann Sums and...
Curated OER
Filling The Urn
Students calculate the area under a curve. In this calculus lesson,students calculate the area by integrating the equation. They calculate the volume of cylindrical shapes created between graphs.
Curated OER
Exploring the Area Under a Curve
Using TI-89, mathematicians explore and investigate Riemann sums to estimate the area under the graphs of intervals by drawing and illustrating rectangles. They review and practice the concepts of summation notation and area formulas to...