Illustrative Mathematics
Illustrative Mathematics: S Cp: False Positive Test Results
A certain test for mononucleosis has a 99% chance of correctly diagnosing a patient with mononucleosis and a 5% chance of misdiagnosing a patient who does not have the infection. Suppose the test is given to a group where 1% of the...
Illustrative Mathematics
Illustrative Mathematics: S cp.a.1, S cp.a.4, and S cp.b.6: The Titanic 1
On April 15, 1912, the Titanic struck an iceberg and rapidly sank with only 710 of her 2,204 passengers and crew surviving. Data on survival of passengers are summarized in the table below. (Data source:...
Illustrative Mathematics
Illustrative Mathematics: N Rn: Operations With Rational and Irrational Numbers
Experiment with sums and products of rational and irrational numbers. Aligns with N-RN.B.3.
Illustrative Mathematics
Illustrative Mathematics: N Rn: Rational or Irrational?
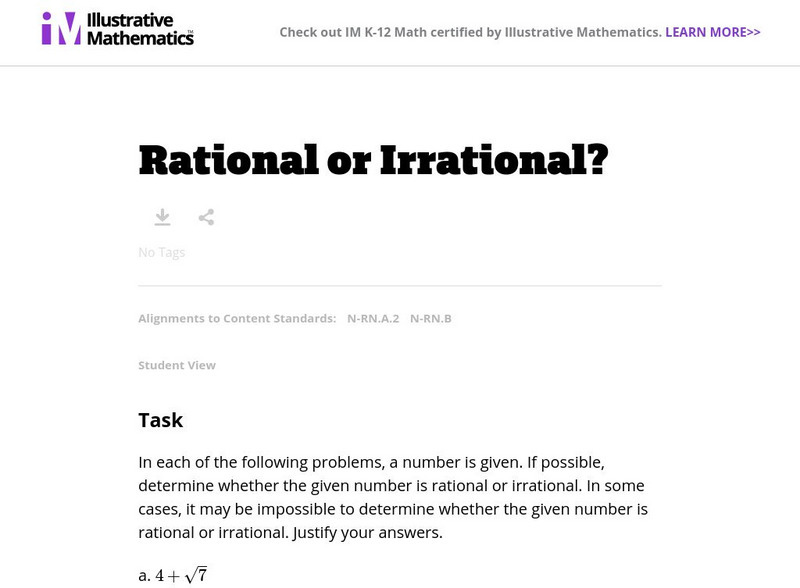
In each of the following problems, a number is given. If possible, determine whether the given number is rational or irrational. In some cases, it may be impossible to determine whether the given number is rational or irrational. Justify...
Illustrative Mathematics
Illustrative Mathematics: N q.a.1: Ice Cream Van
The purpose of this task is to engage students, probably working in groups, in a substantial and open-ended modeling problem. Students will have to brainstorm or research several relevant quantities, and incorporate these values into...
Illustrative Mathematics
Illustrative Mathematics: N q.a.1: Selling Fuel Oil at a Loss
This task is a modeling problem which ties into financial decisions faced routinely by companies. Students will try to keep a balance between maintaining their oil inventory and raising short-term capital for investment or re-investment...
Illustrative Mathematics
Illustrative Mathematics: N q.a.1: Runners' World
This task provides students with an opportunity to engage in Standard for Mathematical Practice 6, attending to precision. It intentionally omits some relevant information. Aligns with N-Q.A.1.
Illustrative Mathematics
Illustrative Mathematics: N rn.a.1: Evaluating Exponential Expressions
The goal of this task is to use properties of exponents for whole numbers in order to explain how expressions with fractional exponents are defined. Aligns with the N-RN.A.1 standard.
Illustrative Mathematics
Illustrative Mathematics: N rn.a.2: Kepler's Third Law of Motion
The purpose of this task is to solve some expressions requiring fractional exponents in the modeling context of planetary motion. Aligns with N-RN.A.2.
Illustrative Mathematics
Illustrative Mathematics: N rn.a.1: Evaluating a Special Exponential Expression
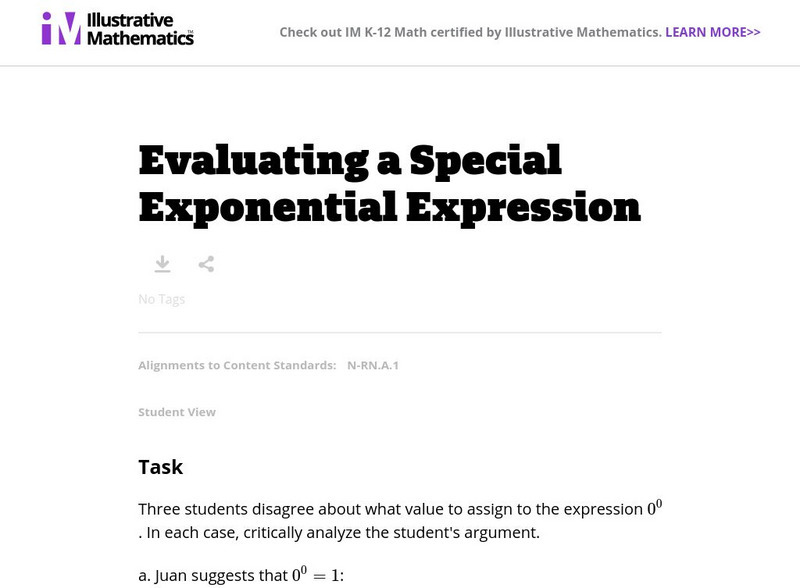
The purpose of this task is to study the rules of exponents in the context of trying to make sense of a challenging mathematical expression. Teachers will critically analyze each student's argument. Aligns with N-RN.A.1.
Illustrative Mathematics
Illustrative Mathematics: S cp.a.3: Lucky Envelopes
This task builds on prior knowledge and understanding of conditional probability, and introduces the concept of independence of events. Multi-colored envelopes are required in this activity. Aligns with S-CP.A.3.
Illustrative Mathematics
Illustrative Mathematics: S cp.b.9: Random Walk Iv
This activity, which follows Random Walk III, is intended for instructional purposes only. The goal for students is to understand how to calculate and compare combinatorial symbols. Aligns with S-CP.B.9.
Illustrative Mathematics
Illustrative Mathematics: S cp.a.2/a.3/a.5 and S cp.b.7: Rain & Lightning
This task uses the same situation to explore different concepts of probability theory. Aligns with the S-CP.A.2.; S-CP.A.3; S-CP.A.5; and S-CP.B.7 standards.
Illustrative Mathematics
Illustrative Mathematics: S cp.b.9: Alex, Mel, and Chelsea Play a Game
Alex, Mel, and Chelsea play a game that has 6 rounds. In each round there is a single winner, and the outcomes of the rounds are independent. For each round the probability that Alex wins is $\frac{1}{2}$, and Mel is twice as likely to...
Illustrative Mathematics
Illustrative Mathematics: G C, G Co Tangent Lines and the Radius of a Circle
In this task, students are shown a circle with center O and point P on the circle. L is a line that is a tangent to the circle at P. They must show that line OP is perpendicular to line L. Aligns with G-C.A.2, G-CO.A, and G-CO.C.9.
Illustrative Mathematics
Illustrative Mathematics: F Tf, G Co, Trigonometric Identities and Rigid Motions
The purpose of this task is to apply translations and reflections to the graphs of the functions f(x)=cos x and g(x)=sin x in order to derive some trigonometric identities. There are also rotations 180 degrees about points on the x-axis...
Illustrative Mathematics
Illustrative Mathematics: G Co Inscribing an Equilateral Triangle in a Circle
Suppose we are given a circle of radius r. The goal of this task is to construct an equilateral triangle whose three vertices lie on the circle. Aligns with G-CO.D.13.
Illustrative Mathematics
Illustrative Mathematics: G Co Construction of Perpendicular Bisector
A and B are two distinct points in the plane and line AB is the segment joining them. The goal of this problem is to construct the perpendicular bisector of segment AB. Aligns with G-CO.D.12.
Illustrative Mathematics
Illustrative Mathematics: G Co Inscribing a Hexagon in a Circle
In this task, students inscribe a regular hexagon inside a circle and compare its area to that of the circle. Aligns with G-CO.D.13.
Illustrative Mathematics
Illustrative Mathematics: G Co Are the Triangles Congruent?
In each of the following diagrams, two triangles are shaded. Based on the information given about each diagram, decide whether there is enough information to prove that the two triangles are congruent. Aligns with G-CO.B.
Illustrative Mathematics
Illustrative Mathematics: G Co Dilations and Distances
The goal of this task is to study the impact of dilations on distances between points. Aligns with G-CO.A.2.
Illustrative Mathematics
Illustrative Mathematics: G Co Fixed Points of Rigid Motions
A rigid motion of the plane is a map of the plane to itself which preserves distances between points. The purpose of this task is to use fixed points as a tool for studying and classifying rigid motions of the plane, i.e., translations,...
Illustrative Mathematics
Illustrative Mathematics: G Co Defining Reflections
The goal of this task is to compare and contrast the visual intuition we have of reflections with their technical mathematical definition. Aligns with G-CO.A.4.
Illustrative Mathematics
Illustrative Mathematics: G Co Defining Rotations
The goal of this task is to encourage students to be precise in their use of language when making mathematical definitions. After working on this problem, they are asked to give a proper definition of a rotation by an angle about a...