Mathematics Vision Project
Module 8: Statistics
What does it mean to be normal in the world of statistics? Pupils first explore the meaning of a normal distribution in the eight-lesson module. They then apply the statistics related to normal distributions such as mean, standard...
West Contra Costa Unified School District
Solving Equations with Variables on Both Sides
So many different ways to solve equations, so little time! Scholars learn how to solve equations with variables on both sides by using several different methods. They apply bar models, decomposition, and traditional algebraic methods to...
West Contra Costa Unified School District
Parallel Lines Cut by a Transversal
Parallel lines seem so right for each other. It's too bad they'll never, ever meet. Learners use tracing paper to discover relationships among angles formed by two parallel lines cut by a transversal. They apply this information to find...
Balanced Assessment
Fermi Area
Discover creative ways to use surface area formulas. In the assessment task, scholars apply estimation strategies to determine surface area in non-routine problems. They consider surface area of kitchen sponges, rock salt crystals, and...
West Contra Costa Unified School District
Polynomial Division
How do you apply the traditional division algorithm to polynomials? Here is an Algebra II lesson that extends the use of the division algorithm to polynomials. After establishing the concept of long division, synthetic division and the...
Balanced Assessment
Local and Global Behavior
Create rules for numerical sequences. Pupils develop local rules and recursive rules for number sequences. The sequences are linear, quadratic, and cubic in nature. Scholars find that some local rules do not work, no matter where in the...
Balanced Assessment
Curvy-Ness
Curves ahead! Develop a numerical measurement of curvy-ness. The class is challenged to come up with a definition of curvy that can be applied to curves. The class members use their defined measurement to describe a curve.
Balanced Assessment
Chameleon Color
Don't let the resource hide from you. In the assessment task, young mathematicians solve problems involving chameleons that change color. Three different problems require individuals to apply parity in various situations.
Balanced Assessment
Bouncing Off the Walls
Apply geometry concepts to improve your pool game! Here scholars create the perfect bank shot using angles of incidence and refraction. They create three different options for the same shot.
Balanced Assessment
Mirror, Mirror II
Apply the concept of similar triangles to design a space in a room. Scholars use similar triangles to determine how a spotlight reflects from a mirror. After drawing the path of the spotlight, individuals find the smallest possible width...
Balanced Assessment
Bicycle Chain II
Apply geometric concepts to a design problem. Individuals examine the structural setup of the chain on a bicycle and use the measurements of the circles to determine the length of the chain.
Mathed Up!
Area of Sector and Length of Arcs
Viewers learn how to apply proportional reasoning to find area of sectors and arc lengths with a video that starts off explaining how to find the areas of circle sectors and the lengths of arcs. Scholars then practice solving problems...
Mathed Up!
Vectors
Young mathematicians connect vectors to geometric shapes by watching a video that expresses vectors in relation to the sides of geometric figures. They then apply what they learned by completing a worksheet of practice questions.
PBL Pathways
Solar Toasters
Help a company maximize their profits! A detailed project-based learning activity examines two production scenarios. Your young scholars write a linear demand function. They then apply the function to develop a revenue, cost, and profit...
Noyce Foundation
Perfect Pair
What makes number pairs perfect? The resource provides five problems regarding perfect pairs of numbers, the definition of which changes in complexity with each task. Solutions require pupils to apply number sense and operations, as well...
Noyce Foundation
Diminishing Return
Challenge individuals to compete as many tasks as possible. Lower-level tasks have pupils apply costs and rates to solve problems. Upper-level tasks add algebraic reasoning and conditional probability to the tasks.
CK-12 Foundation
Matrices to Represent Data: Houndstooth
Apply matrices to fashion. Here your classes use a matrix to create a popular clothing design. As they construct the pattern, they review the dimensions of a matrix by considering the rows and columns.
CK-12 Foundation
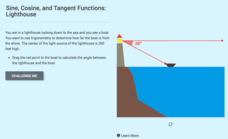
Sine, Cosine, and Tangent Functions: Lighthouse
How far is that boat from the lighthouse? Scholars create diagrams to represent a scenario given the angle of depreciation from a lighthouse to a boat. Learners apply the basic trigonometric functions to find various distances stemming...
CK-12 Foundation
Pythagorean Theorem to Determine Distance: Neighborhood Map
Find the distance between various locations in a neighborhood. Scholars use the interactive to find distances between locations on a map. The map is overlaid onto a grid to provide coordinates for each location, and pupils apply...
CK-12 Foundation
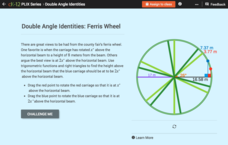
Double Angle Identities: Ferris Wheel
Use a Ferris wheel to soar to new heights of understanding on double angle identities. Here is an interactive that applies an example of a Ferris wheel to show how doubling the angle does not double the value of a trigonometric ratio....
CK-12 Foundation
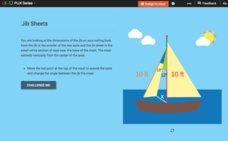
Determination of Unknown Triangle Measures Given Area: Jib Sheets
Solving triangles is a breeze. Young boat enthusiasts solve problems involving triangles in the context of sails on a boat. They must apply different strategies, including the Law of Cosines and area formulas.
CK-12 Foundation
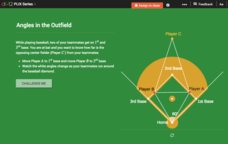
Law of Cosines: Angles in the Outfield
Take me out to the trig class. Individuals use an interactive to see how angles on a baseball field change as the position of two runners change. A set of challenge questions has them apply the Law of Cosines to solve problems.
CK-12 Foundation
Angle-Angle-Side Triangles: Garden Gate
Good fences make good gardens. Individuals use an interactive to see how angles and sides relate in a triangular-shaped garden fence. They apply the Law of Sines to find the length of the garden gate (third side of triangle) given two...
CK-12 Foundation
Distance Between Two Polar Coordinates: Exploring Changes in Angle and Radius
Get straight answers on a curved grid. An interactive has learners apply the Law of Cosines to find the distance between two points on the polar coordinate plane. The pupils use the radii lengths and the angle between the two radii to...
Other popular searches
- Grade 9 Applied Math
- Applied Math Weight
- Applied Math Blueprint
- Applied Math 20
- Worksheets Applied Math
- Applied Mathematics
- Applied Math Parallel
- Applied Math Electricity
- Applied Math Exam
- Applied Math for Aviation
- Applied Math Store
- Applied Math Conservation