EngageNY
Mid-Module Assessment Task - Algebra 1 (module 4)
Performance task questions are the most difficult to write. Use this assessment so you don't have to! These questions assess factoring quadratics, modeling with quadratics, and key features of quadratic graphs. All questions require...
EngageNY
Translations
Learn through constructions! Learners examine a translation using constructions and define the translation using a vector. Pupils then construct parallel lines to determine the location of a translated image and use the vector as a guide.
EngageNY
End-of-Module Assessment Task - Algebra 1 (Module 1)
What do your young geniuses really know? Assess the procedural knowledge of your pupils at the same time as their higher-level thinking with an assessment that identifies their depth of knowledge. Topics include solving...
EngageNY
Mid-Module Assessment Task: Pre-Calculus Module 5
Determine if any reteaching with a mid-module assessment task. The assessment covers the general multiplication rule, permutations and combinations, and probability distributions for discrete random variables.
EngageNY
Graphs of Simple Nonlinear Functions
Time to move on to nonlinear functions. Scholars create input/output tables and use these to graph simple nonlinear functions. They calculate rates of change to distinguish between linear and nonlinear functions.
EngageNY
Basic Properties of Similarity
Does the symmetry and transitive property apply to similarity? The 10th segment in a series of 16 presents the class with a group of explorations. The explorations have pairs show that similarity is both symmetrical and transitive. It...
EngageNY
Properties of Trigonometric Functions
Given a value of one trigonometric function, it is easy to determine others. Learners use the periodicity of trigonometric functions to develop properties. After studying the graphs of sine, cosine, and tangent, the instructional...
EngageNY
Tangent Lines and the Tangent Function
Construct tangent lines and make the connection to tangent functions. An informative activity reviews the geometry origins of the tangent function. Pupils use that information to determine how to construct a tangent to a circle from a...
EngageNY
Law of Cosines
Build upon the Pythagorean Theorem with the Law of Cosines. The 10th part of a 16-part series introduces the Law of Cosines. Class members use the the geometric representation of the Pythagorean Theorem to develop a proof of the Law of...
EngageNY
Modeling with Inverse Trigonometric Functions 1
Where should I stand to get the best view? Pupils use inverse trigonometric functions to determine the horizontal distance from an object to get the best view. They round out the lesson by interpreting their answers within context.
EngageNY
Examples of Dilations
Does it matter how many points to dilate? The resource presents problems of dilating curved figures. Class members find out that not only do they need to dilate several points but the points need to be distributed about the entire curve...
EngageNY
Dilations on the Coordinate Plane
Dilations from the origin have a multiplicative effect on the coordinates of a point. Pupils use the method of finding the image of a point on a ray after a dilation to find a short cut. Classmates determine the short cut of being able...
EngageNY
Mid-Module Assessment Task: Grade 8 Module 6
Make sure pupils have the skills to move on to the second half of the module with a mid-module assessment task. The formative assessment instrument checks student learning before moving on to the rest of the lessons in the unit.
EngageNY
Mid-Module Assessment Task: Grade 8 Module 1
Assess your young mathematicians' knowledge and understanding of the properties of exponents. The questions in the seventh lesson of 15 incorporate the properties learned in the first six modules of this series. Individuals use and apply...
EngageNY
End-of-Module Assessment Task: Grade 8 Module 6
Test your knowledge of linear functions and models. The last installment of a 16-part module is an end-of-module assessment task. Pupils solve multi-part problems on bivariate data based on real-world situations to review concepts from...
EngageNY
Efficacy of Scientific Notation
How many times could California fit into the entire United States? Pupils use scientific notation to find the answer to that question in the 12th installment of 15 lessons. It asks scholars to write numbers in scientific notation and...
EngageNY
End-of-Module Assessment Task: Grade 8 Module 1
It's all in the numbers. Determine your pupils' level of understanding of scientific notation using this assessment task. The final lesson in the series assesses scholars on the application of scientific notation in real-life situations....
EngageNY
Characteristics of Parallel Lines
Systems of parallel lines have no solution. Pupils work examples to discover that lines with the same slope and different y-intercepts are parallel. The 27th segment of 33 uses this discovery to develop a proof, and the class determines...
EngageNY
Mid-Module Assessment Task: Grade 8 Module 4
Determine what the class knows about linear equations. The three-question mid-module assessment is segment 15 in a 33-part series. The assessment includes writing and solving one-variable linear equations and graphing proportional...
EngageNY
Sequencing Rotations
Discover the result of a sequence of rotations about different centers. Pupils perform rotations to examine the patterns. They also describe the sequence of rotations that performed to reach a desired result in the ninth installment in a...
EngageNY
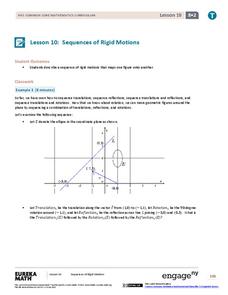
Sequences of Rigid Motions
Examine the various rigid transformations and recognize sequences of these transformations. The lesson asks learners to perform sequences of rotations, reflections, and translations. Individuals also describe a sequence that results in...
EngageNY
The Line Joining Two Distinct Points of the Graph y=mx+b Has Slope m
Investigate the relationship between the slope-intercept form and the slope of the graph. The lesson plan leads an investigation of the slope-intercept equation of a line and its slope. Pupils realize the slope is the same as the...
EngageNY
More on the Angles of a Triangle
Angles and triangles: they're all connected. Uncover the connections between angles in triangles. Scholars learn how to find both exterior and interior angle measures in triangles. The instructional activity emphasizes the vocabulary...
EngageNY
Examples of Functions from Geometry
Connect functions to geometry. In the ninth installment of a 12-part module, young mathematicians create functions by investigating situations in geometry. They look at both area and volume of figures to complete a well-rounded lesson.
Other popular searches
- Calculus Derivatives
- Derivative Nouns
- Calculus, Derivatives
- Base Word Derivatives
- Complex Derivative
- Derivatives of Logarithms
- Second Derivative
- Derivative Quotient Rule
- Word Derivatives
- Derivative Function
- Directional Derivatives
- Latin Derivatives