Illustrative Mathematics
Illustrative Mathematics: N q.a.1: Fuel Efficiency
The problem requires learners to not only convert miles to kilometers and gallons to liters but they also have to deal with the added complication of finding the reciprocal at some point. Aligns with N-Q.A.1.
Illustrative Mathematics
Illustrative Mathematics: N q.a.1: How Much Is a Penny Worth?
Students will determine the "real worth" of a penny by analyzing the present worth of the various metals that are used to compose the penny. Aligns with N-Q.A.1.
Illustrative Mathematics
Illustrative Mathematics: N q.a.1, a ced.a.1, and N q.a.2: Harvesting the Fields
This task is about farm-workers who are harvesting two fields. Students are presented with a scenario involving two teams of workers, two different sizes of fields, and work spread out over two days. They must calculate how many...
Illustrative Mathematics
Illustrative Mathematics: N cn.c.7 and a rei.b.4.a: Completing the Square
The goal of this task is to solve quadratic equations with complex roots by completing the square. Aligns with N-CN.C.7 and A-REI.B.4.aI.
Illustrative Mathematics
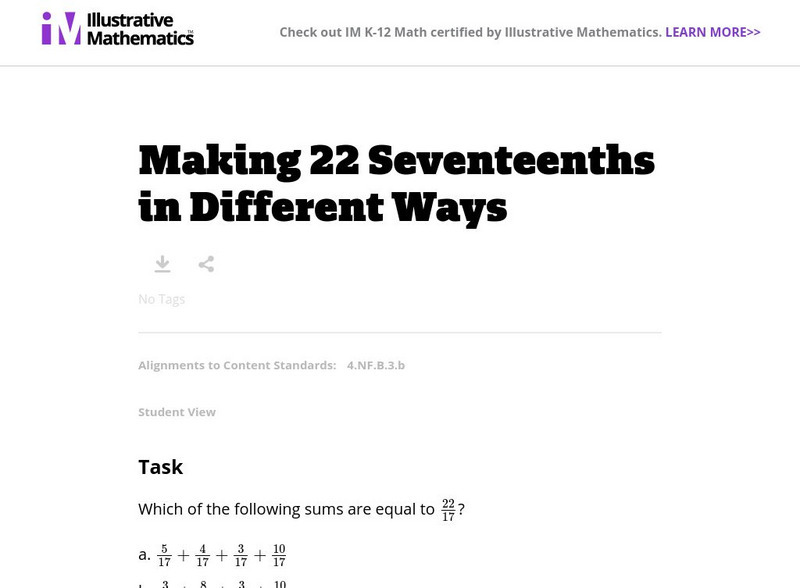
Illustrative Mathematics: 4.nf Making 22 Seventeenths in Different Ways
This task is a straightforward task related to adding fractions with the same denominator. The main purpose is to emphasize that there are many ways to decompose a fraction as a sum of fractions, similar to decompositions of whole...
Illustrative Mathematics
Illustrative Mathematics: 4.g Lines of Symmetry for Circles
Mr. Watkins asked his students to draw a line of symmetry for a circle with center $O$ pictured below: Aligns with 4.G.A.3.
Illustrative Mathematics
Illustrative Mathematics: 3.nf Find 7/4 Starting From 1, Assessment Variation
Students find 7/4 starting from 1. Aligns with 3.NF.A.2; CCSS.Math.Content.3.NF.A.2.a Represent a fraction 1/b on a number line diagram
Illustrative Mathematics
Illustrative Mathematics: A apr.c.5 and a apr.a.1: Powers of 11
This task has students combine polynomial arithmetic with pattern-matching. Aligns with A-APR.C.5 and A-APR.A.1.
Illustrative Mathematics
Illustrative Mathematics: A sse.b.3.a and a sse.a.2: Cubic Identity
This task presents a challenging exercise in both algebraic manipulations and seeing structure in algebraic expressions. Aligns with A-SSE.B.3.a and A-SSE.A.2.
Illustrative Mathematics
Illustrative Mathematics: A ced.a.2: Throwing a Ball
Using a ball thrown vertically as the real life scenario, this task has a couple of aspects designed to encourage students to attend to the structure of a given equation and the meaning of the variables in it. Aligns with HSA-CED.A.2.
Illustrative Mathematics
Illustrative Mathematics: A ced.a.4: Rewriting Equations
The goal of this task is to manipulate equations in order to solve for a specified variable. Aligns with HSA-CED.A.4.
Illustrative Mathematics
Illustrative Mathematics: A apr.b.3 and a apr.b.2: Graphing From Factors Iii
The task has students use the remainder theorem to deduce a linear factor of a cubic polynomial, and then to completely factor the polynomial. Aligns with A-APR.B.3 and A-APR.B.2.
Illustrative Mathematics
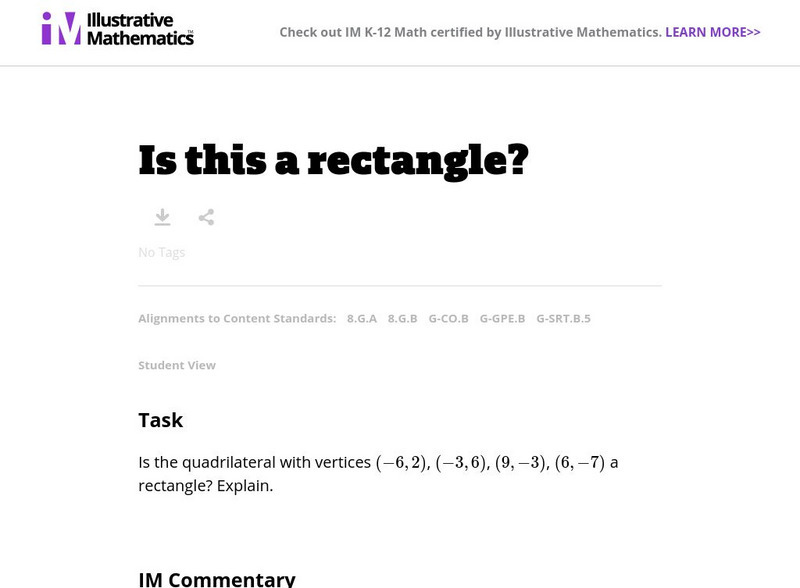
Illustrative Mathematics: 8.g, G Gpe, G Srt, G Co Is This a Rectangle?
For this task, students are given four sets of coordinate pairs and are asked to determine if they represent a rectangle. Aligns with 8.G.A, 8.G.B, G-CO.B, G-GPE.B, and G-SRT.B.5.
Illustrative Mathematics
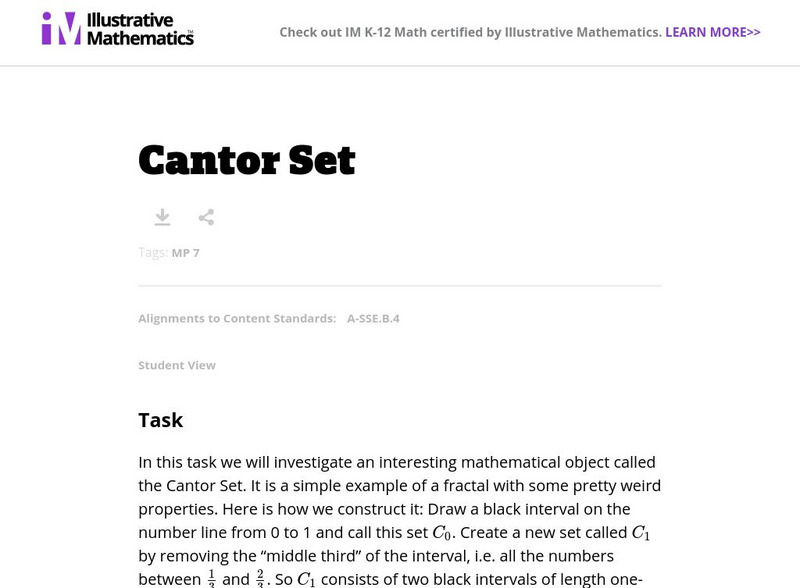
Illustrative Mathematics: A sse.b.4: Cantor Set
This tasks requires students to explore properties of the Cantor Set, which has many uncountable points, no intervals of real numbers, and has length of zero. Aligns with A-SSE.B.4.
Illustrative Mathematics
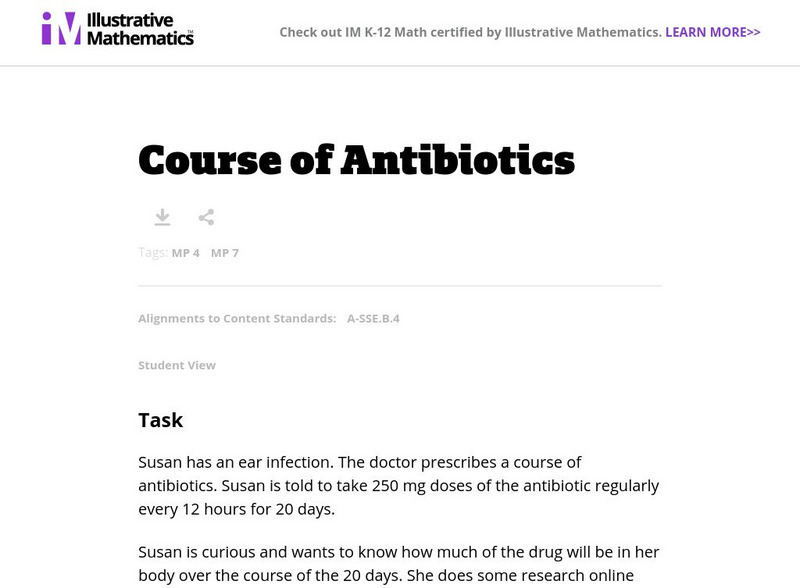
Illustrative Mathematics: A sse.b.4: Course of Antibiotics
This task presents a real world application, prescribing a course of antibiotics. In this task, a finite geometric series is utilized. Aligns with A-SSE.B.4.
Illustrative Mathematics
Illustrative Mathematics: F if.c.7.a, a sse.b.3, F if.c.7: Graphs of Quadratic
During this task, learners will realize that equivalent expressions produce the same graph. The lesson will lead to discussions about the value of different forms of equations. Aligns with F-IF.C.7.a, A-SSE.B.3, and F-IF.C.7.
Illustrative Mathematics
Illustrative Mathematics: A sse.b.4: A Lifetime of Savings
The purpose of this instructional task is to give young scholars an opportunity to construct and find the value of a geometric series in a financial literacy context. Aligns with A-SSE.B.4.
Illustrative Mathematics
Illustrative Mathematics: A sse.a.1.b and a sse.b.3 Increasing or Decreasing? 2
The purpose of this task is to help students see manipulation of expressions as an activity undertaken for a purpose. Aligns with A-SSE.B.3.
Illustrative Mathematics
Illustrative Mathematics: A sse.a.2: Equivalent Expressions
This problem expects students to choose an operation in response to a question about structure. A-SSE.A.2.
Illustrative Mathematics
Illustrative Mathematics: A sse.b.3: Ice Cream
This task illustrates the process of rearranging the terms of an expression to reveal different aspects about the quantity it represents. Aligns with A-SSE.B.3.
Illustrative Mathematics
Illustrative Mathematics: A sse.b.3.c Forms of Exponential Expressions
This task can be used for assessment, practice or even to motivate learners via an in-class discussion of why it is useful to rewrite exponential expressions in equivalent forms. Aligns with A-SSE.B.3.c.
Illustrative Mathematics
Illustrative Mathematics: A sse.b: Taxes and Sales
This task is not about computing the final price of the shirt but about using the structure in the computation to make a general argument. Aligns with A-SSE.B.
Illustrative Mathematics
Illustrative Mathematics: A sse.b.3.b: Rewriting a Quadratic Expression
The goal of this task is to complete the square in a quadratic expression in order to find its minimum or maximum value. Aligns with HSA-SSE.B.3.b.
Illustrative Mathematics
Illustrative Mathematics: A Sse.4: You Tube Explosion
This task, commentary, and solutions, were authored by Lacey Dixon and Jonathan Utegaard of The University of Arizona. The purpose of this task is to have students derive the formula for the sum of a specific finite geometric series....